
The first difference is found by subtracting each term from the following term: Recursive Formula Sequence (first five terms) Explicit Formula Online math. This is a sequence with a constant second difference. Converting Metric Measures F G to E 35 36 Triangles, Quadrilaterals & Other. Please provide additional context, which ideally explains why the question is relevant to you and our community.Some forms of context include: background and motivation, relevant definitions, source, possible strategies, your current progress, why the question is interesting or important, etc. The formula which you give, $a_n=\frac=a_n+n+1$. It seems to have a non-standard procedure to it.
CONVERTING RECURSIVE SEQUENCE TO EXPLICIT EQUATION HOW TO
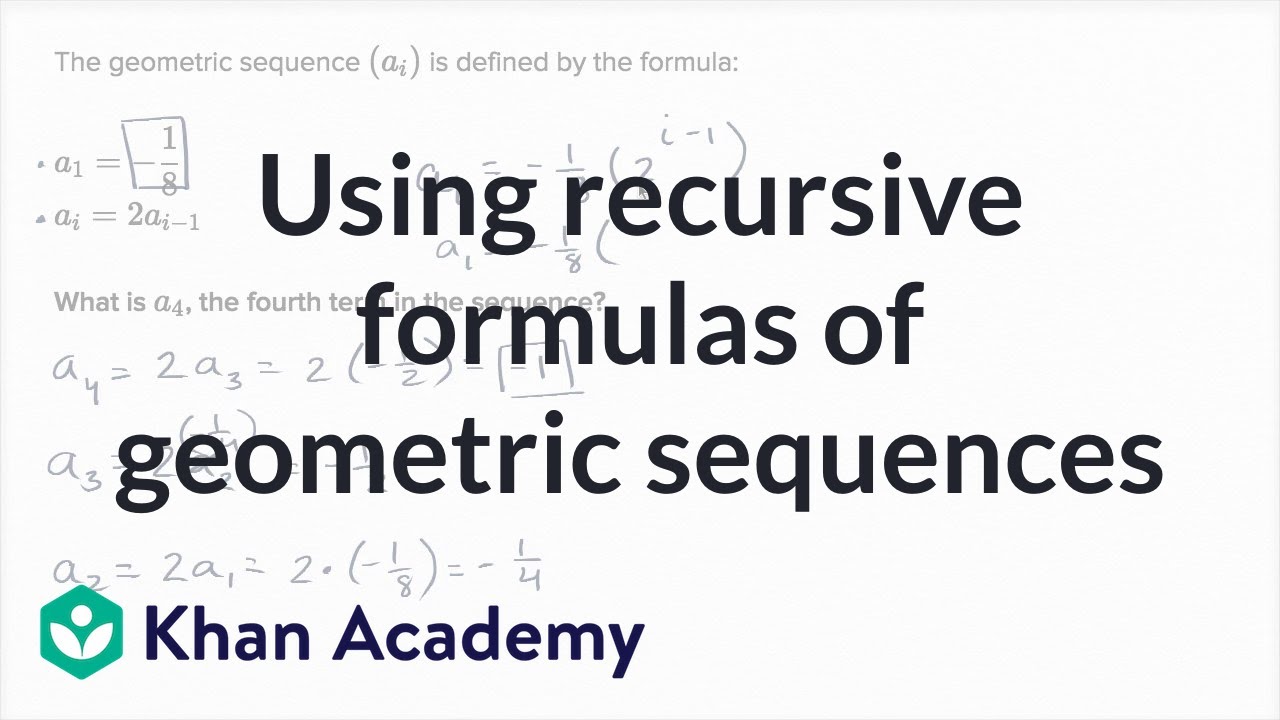
That means the problem is me not knowing how to deal with that problem in the first place, I think. I'm sure I didn't mess up the system of equations or any step since I used automated equation solving to make sure. As you can see, this final conversion is. Questions Tips & Thanks Want to join the conversation Sort by: Top Voted James K 10 years ago At 0:25, why does x have to be a positive integer ( 10 votes) Upvote Flag ray. Find the recursive formula of the sequence. Recall that the recursive formula gives us two pieces of information. Sal solves the following problem: The explicit formula of a geometric sequence is g (x)98 (x-1). Lets find a recursive formula for the sequence. then plugging in known $n$ and $a_n$ values to get a system of equations and then finally plug in the resulting $\alpha$ and $\beta$ to get the explicit formula, which however turned out to be complete gibberish. Learn how to convert between recursive and explicit formulas of arithmetic sequences. which is the standard procedure I've been taught, then solving for $r$ to get $r_1$ and $r_2$ and adding $\alpha$ and $\beta$ like I've been taught to get: To solve this, I've tried converting it to:Īnd treating n as the superscript of r. The correct explicit formula that I don't know how to get to for this is: The sequence this is supposed to represent is: $0, 2, 5, 9, 14, 20, 27. As an example, consider this recursive function definition, which defines the Collatz sequence: f(1) 0 f(2n) 1 + f(n) f(2n + 1) 1 + f(6n + 4) It's not known whether or not this is even a well-defined function or not.

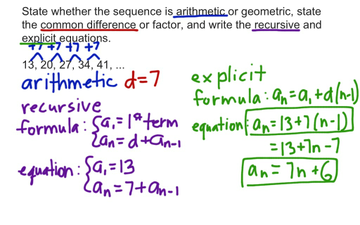
However my problem is I've encountered a type of this problem I haven't been thought how to approach, and can't find the solution anywhere: In general, there is no algorithm for converting a recursive form into an iterative one. So, I know how to convert to explicit formulas things like the Fibonacci sequence cause it only consists of $a_n$ like this:


 0 kommentar(er)
0 kommentar(er)
